Ernest Lawrence
An Absolute Smasher

Ernest Lawrence
Smashing
The Older Tradition
In the good old days - say 1910 - if you wanted to bombard an atomic nucleus with sub-atomic particles, nothing was easier. You just got a lump of beeswax, drilled a hole into it, and stuck in a bit of radium - surprisingly available at the time. The stick a piece of metal over the hole. The beeswax stops the particles from the radium - which are simply the nucleus of a helium atom - and so the particles can't do anything else except strike the metal. After days, weeks, or months, you'd hand the metal over to an analytical chemist, and he'd tell you if you had created a new element.
Or you could mix the radium with beryllium and the particle shooting out would be a neutron. You could make even more elements with neutrons since they could go into a nucleus easier than alpha particles. These beeswax contraptions were called particle "howitzers", and up into the 1920's this was pretty much the way nuclear transformations were studied.
There are, though, only so many elements, and it didn't talk long to more or less exhaust the options. That is, there were no more elements to transmute.
At that point, a young physics professor at Berkeley - a Midwesterner from South Dakota - had an idea. Ernest Lawrence had been lured away from Yale to the West Coast since Berkeley had told him that he would have more time and resources for research. The idea of not having to teach as many undergrads seemed like a good idea and particularly there were assurances that Ernest would be able to collaborate with professors of other departments.
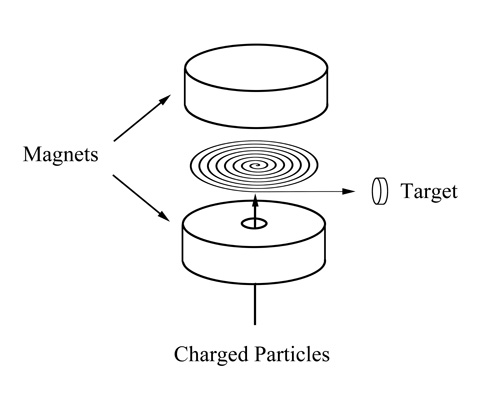
Electrostatics tells us that if you sent a charged particle through a magnetic field, it would accelerate. The acceleration would produce a more energetic particle and it should be able to smack into a nucleus with more energy than a measly particle howitzer could. This would produce new reactions. Better still, since you could increase the magnetic field using electromagnets, there was no real limit to how much you could energize the particles in the "accelerator". So you - at least in principle - have an infinite number of things to do.
The difficulty was that the more energy you wanted, then the longer the path the particle traveled had to be. But there were limits to the size of the labs.
But acceleration doesn't just mean changing speed. It can also mean changing direction. And if you sent a charged particle perpendicular to the magnetic field - that is, send it between two flat magnets - the particle not only speeds up but begins to move in spirals. So in a relatively small space, the particle can travel quite a ways and pick up a lot of speed.
Ernest's first cyclotron - as we call it - could be held in one hand and was almost literally put together with spit and sealing wax.
After a while, though, everyone noticed that it was getting harder and harder to make the protons go faster. They weren't going as fast as the magnetic field should push them.
The problem though was Albert Einstein. Or rather what Albert had discovered. And that's as things speed up, they get heavier.
Or rather they get more massive. And if something is more massive, it gets harder to push faster.
Now it's easy enough to prove that in relativity, if something moves faster it's time slows down and the distance it travels is shorter.
Middle school math works with the mass as well, but the derivation a bit more lengthy.
But we'd still like to see how it's done.
I thought you would, as Captain Mephisto said to Sidney Brand. It's very simple really.
First of all, remember that velocity of a particle is the speed divided by the distance.
| v | = |
|
Or more exactly, velocity is the difference of distance with difference in time:
| v | = |
|
Which if the particle isn't moving at a constant speed means you have the resort to calculus and use the derivative of distance withe time:
| v | = |
|
Now this is the velocity as measured from a reference frame that isn't moving. That is we are measuring the velocity from a stationary reference frame.
But according to Albert - and also Heinrich Lorentz - if you're measuring the velocity from a different reference frame - one that is moving - you have to make a relativistic correction:
| x | = |
|
... where v is the velocity of the moving reference frame.
|
= |
|
References
Books
Big Science: Ernest Lawrence and the Invention that Launched the Military-Industrial Complex, Michael Hiltzik, Simon and Schuster, 2015.
Internet Resources
Lawrence and the Cyclotron, American Institute of Physics Web History
"Nuclear Physics in the 1930's"
"The First Cyclotrons"
"Lawrence and the Bomb"
Winners Science, http://winnerscience.com/. This website has a number of straightforward algebraic derivations devoid of the incomprehensibilities often found at other sites that - quote - "explain" - unquote science. You don't - believe it or not - have to write at the advanced graduate or research level to explain things.
"Variation of Mass with Velocity and Its Derivation"Winners Science, October 10, 2011.
"Relativistic Addition of Velocity", Winners Science, October 7, 2011.
Return to Abraham Robinson Caricature