(Click image to zoom in.)
In which by using modern technology and literary devices, various details are given for systematic speculation in what Julius might have called the ludi fortium and in which are discussed certain apparent paradoxes in the hope of furthering the cause of truth, justice, and the American pocketbook and losing your shirt in a rational manner.
Everyone knows that Arnold Rothstein (1882 - 1928), known to his many acquaintances as "The Brain", was famous for his "bankrolling". That is, if you needed some dough for some - ah - "enterprise", you'd go up to Arnold and explain your needs. He'd reach into his pocket and pull out a thick roll of bank notes and hand it over. Of course, you had to pay Arnold back and at interest1, but that was the way Arnold did business.
So suppose we have the following - and purely literary - scenario. Arnold was going about his business - as we see above with his friends Alvin "Titanic" Thompson and George "Hump" McManus - when he ran into an old acquaintance named Gamblin' Joe Blow.
You see, Gamblin' Joe considered himself something of a mathematical genius, and he was able to construct a copy of Charles Babbage's "difference engine". With the "engine", Joe came up with a strategy for betting in various games of chance.

Charles
He invented the engine.
On the first bet, Joe said, you you'd bet the minimum allowed (all gambling tables have a minimum). Say it was a $1 bet on red at the roulette wheel. Then if you won you'd pocket your winnings and again bet the minimum.
But if you lost, you double the previous bet. That way if you lost your first bet, the next bet would be $2. If you then won, with the $1 lost on the previous bet and your $2 win, you would be ahead by $1. You'd then go back and bet the minimum. You would continue with this betting sequence, playing the minimum after a win and doubling your bet after a loss.
It was impossible, Joe claimed with a smile, for him to lose. By doubling your last bet each time your lose, once you won then you would wipe all previous losses and increase your bankroll by $1.
Since you're betting red, Gamblin' Joe nodded - remember he was a mathematical whiz - you'll win 47% of the time. Therefore you'll have a net gain of $1 every 2.1 spins of the wheel. And as seeing is believing, Joe provided a graph of what he calculated to be the expected wins.
Expected Wins of
Joe's "Double Up" Method
So at the start of a game, your bets might run like:
Sample Play |
|||||
| Spin | Bet ($) | Outcome | Winnings per Spin | Net Winnings | Comments |
| 1 | 1 | Win | +1 | +1 | Ha! Won! |
| 2 | 1 | Win | +1 | +2 | Won Again! |
| 3 | 1 | Lose | -1 | +1 | Lost! Double Next Bet! |
| 4 | 2 | Lose | -2 | -1 | Lost! Double Next Bet! |
| 5 | 4 | Lose | -4 | -5 | Lost Again! Double Next Bet! |
| 6 | 8 | Win | +8 | +3 | Won! So Recouped Losses And Are $1 Above Last High (Spin 2) |
| 7 | 1 | Win | +1 | +4 | Back to the $1 Bet. |
Sure enough, with each win your bankroll increases by $1.
Of course, Joe pointed out that there were streaks of bad luck. But even if you lost 6 times in a row that would only be $63. So if Arnold could supply the "ready" to cover such losses, once Joe won the next spin Arnold could get his dough back and Joe would show a profit.
Once more to emphasize his argument Joe used the difference engine with what are known as resampling or "bootstrap" statistics to plot out a - quote - "typical run" - unquote. But even with a string of losses, Joe claimed you should keep making money.
Joe's Bootstrap Graph
So what he'd need, Joe said, was enough of a bankroll to cover any early losing streaks. Then after a while Joe claimed he would have earned enough so he would have a cushion against future losses. If Arnold could hand over a good stake, say a grand, Joe said he should be able to win - as the mathematicians say - in an increasing monotonic linear manner.
"Nihil Sub Sole Novum"
- Arnold Rothstein Ecclesiastes 1:9
Of course, with his own experience Arnold immediately recognized Joe's - quote - "amazing betting method" - unquote - was nothing new. It was, in fact, simply the well-known Martingale System. The Martingale System is one of the oldest "progressive" gambling systems and every gambler knew about it. But Arnold liked Joe's moxie and handed him the grand.
In any case fortified with his system (and Arnold's grand) Joe hied over to Bugsy and Virginia's Gambling Casino. He quickly found a table with a $1 minimum and began to play.2 Naturally he kept tabs on his winnings.
Footnote
Actually in Arnold's time, Joe could probably find cheaper wheels. Even into the 1960's there were the "downtown" casinos on Las Vegas's Fremont Street that had roulette tables with a dime minimum.
Bugsy and Virginia.
Although Joe's increase was modest, for the first hour and a half he saw a fairly steady increase.
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) | Comments |
| - | - | - | - | 1000 | Starting Off |
| 1 | 1 | WIN | 1 | 1001 | Won! |
| 2 | 1 | LOSE | -1 | 1000 | First Loss So Double Up |
| 3 | 2 | WIN | 2 | 1002 | Won Again! So Good |
| 4 | 1 | LOSE | -1 | 1001 | Second Loss Double Up Again. |
| : : | : : | : : | : : | : : | Keep Playing! |
| 47 | 1 | WIN | 1 | 1024 | Showing Profit |
| 48 | 1 | LOSE | -1 | 1023 | Humph. |
| 49 | 2 | LOSE | -2 | 1021 | Lost a Couple of Spins |
| 50 | 4 | WIN | 4 | 1025 | But Still Looks Good |
| 51 | 1 | LOSE | -1 | 1024 | Lost! |
| 52 | 2 | LOSE | -2 | 1022 | Lost Again! |
| 53 | 4 | LOSE | -4 | 1018 | Hm. Three Losses in a Row |
| 54 | 8 | LOSE | -8 | 1010 | Four? |
| 55 | 16 | LOSE | -16 | 994 | Shoot! Five in a Row |
| 56 | 32 | WIN | 32 | 1026 | Whew! Won Again Finally! |
| 57 | 1 | LOSE | -1 | 1025 | Can't Win 'Em All |
| 58 | 2 | LOSE | -2 | 1023 | Couple Of Losses Big Deal! |
| 59 | 4 | WIN | 4 | 1027 | Won Again! S'Awright and a Net Increase. |
So things still seemed to be working.
"The world of reality has its limits, the world of imagination is limitless"
- Jean-Jacques Rousseau
But suddenly Joe found himself loudly and teemingly expressing surprise at what happened next. He began loosing - a lot.
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) | Comments |
| : : | : : | : : | : : | : : | : : |
| 59 | 4 | WIN | 4 | 1027 | Previous Winning Spin |
| 60 | 1 | LOSE | -1 | 1026 | Lost Once! |
| 61 | 2 | LOSE | -2 | 1024 | Lost Twice! |
| 62 | 4 | LOSE | -4 | 1020 | Lost Thrice! |
| 63 | 8 | LOSE | -8 | 1012 | Quadrice! |
In fact, he kept on losing until his brankroll dropped to $900.
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) | Comments |
| 64 | 16 | LOSE | -16 | 996 | Quince! |
| 65 | 32 | LOSE | -32 | 964 | Sence! |
| 66 | 64 | LOSE | -64 | 900 | Sheesh! Whaddaya do now? |
Now he was $100 short of his initial bankroll! That wasn't according to plan.
Well, since he lost on his last bet all he could do was to double up. As his last bet was $64 he now laid down $128.
But then Joe came face to face with the reality of casino gambling:
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) | Comments |
| 67 | 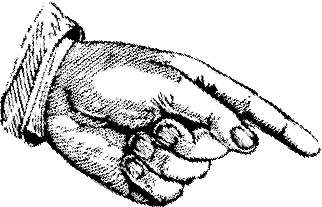 128 128 | - | - | 900 | ERROR! ERROR! |
Instead of covering the bet, the dealer turned to Joe more in sorrow than in anger.
"Sorry, buddy," he said. "You can only bet $100. That's the limit at this table."
Yes, all casino bets have not just minimum bets but betting MAXIMUMS.3
Footnote
The traditional plurals are maxima and minima. But dictionaries do list maximums and minimums as acceptable alternatives.
Despite what it may seem maximums are not large corsages worn with evening gowns.
So here's Joe's dilemma. If he bets the $100 and wins, he'll still be $28 short of recouping his losses. So the idea that on each win you'll always increase the previous high bankroll by $1 isn't correct.
Well, at least if Joe wins, he'll be back with his original $1000 bankroll. So he lays $100 on red.
But Joe's (bad) luck continues:
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) | Comments | 66 | 64 | LOSE | -64 | 900 | Doubling would need $128 |
67 | 100 | LOSE | -100 | 800 | But $100 Is the Maximum |
68 | 100 | LOSE | -100 | 700 | Dang! | 69 | 100 | LOSE | -100 | 600 | Dang! | 70 | 100 | LOSE | -100 | 500 | Dang! |
At this point, Joe paused - not for refreshment - but to plot out his wins and losses.
Joe's First Session
(Continuing)
Hm. After 70 spins - two hours at the table - far from seeing his steadily monotonic linear increase in winnings, Gamblin' Joe has lost a bundle - half his (or rather Arnold's) bankroll.
But at least Joe still had money and so he kept betting and - thankfully - on the next spin he won.
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) | 71 | 100 |  WIN WIN | 100 | 600 |
But now he found himself in yet another dilemma. There's no way a single bet will recoup his losses. So there are two ways to proceed. He could keep betting the maximum until he recoups his losses. But this way in five rolls he might lose everything.
Or he could start the betting sequence again starting out with his $600 bankroll. That is, he would first bet $1 and then when he wins he'll again bet $1 and double up if he looses. Prudence dictated this more economical option and thankfully Joe's bankroll began to slowly climb back up.
Joe's New Session
(Starting Afresh)
So after another six or so hours at the wheel, Joe's bankroll has upped to over $670.
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) |
| : | : | : | : | : |
| 268 | 4 | WIN | 4 | 669 |
| 269 | 1 | WIN | 1 | 670 |
| 270 | 1 | LOSE | -1 | 669 |
| 271 | 2 | LOSE | -2 | 667 |
| 272 | 4 | WIN | 4 | 671 |
| 273 | 1 | WIN | 1 | 672 |
Perhaps with just a couple of more days at the wheel, Joe will be back at $1000.
And indeed Joe's luck continued. His bad luck, that is.
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) |
| : | : | : | : | : |
| 268 | 4 | WIN | 4 | 669 |
| 269 | 1 | WIN | 1 | 670 |
| 270 | 1 | LOSE | -1 | 669 |
| 271 | 2 | LOSE | -2 | 667 |
| 272 | 4 | WIN | 4 | 671 |
| 273 | 1 | WIN | 1 | 672 |
| 274 | 1 | LOSE | -1 | 671 |
| 275 | 2 | LOSE | -2 | 669 |
| 276 | 4 | LOSE | -4 | 665 |
| 277 | 8 | LOSE | -8 | 657 |
| 278 | 16 | LOSE | -16 | 641 |
| 279 | 32 | LOSE | -32 | 609 |
| 280 | 64 | LOSE | -64 | 545 |
| 281 | 100 | LOSE | -100 | 445 |
| 282 | 100 | LOSE | -100 | 345 |
| 283 | 100 | LOSE | -100 | 245 |
| 284 | 100 | LOSE | -100 | 145 |
| 285 | 100 | LOSE | -100 | 45 |
Now what does he do? He can't even bet the maximum - $100. And if he bets all he has left and wins he'll only have $90. And if he looses he'll be wiped out.
And sure enough the last spin is:
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) |
| 285 | 45 | LOSE | -45 | 0 |
Joe now looked at his progress ...
Joe's Final Session
... and checked when the next boat to Rio sails.
"The roulette table pays nobody except him that keeps it."
- George Bernard Shaw, Maxims for Revolutionists
Curmudgeons, of course, will point out all this brouhaha is merely a fictitious and contrived example aimed at warning bettors away from gambling systems. After all, a single example does not general principals dictate.
Instead what if Joe had a lot of partners all loaded up with $1000 bankrolls? And let the examples be dictated by the true random spins according to the odds of roulette. Only then can we see how such a betting system really fares.
That is a reasonable request and can be realized using the modern equivalent of Charles's difference engine. So we'll have Joe team up with Bob, Freda, Henry, Mia, Willow, George, Harry, Olivia, Florence, Joseph, Leo, Archie, Oscar, Isla, Ivy, Arthur, Noah, Lily, Ava, Oliver, Alexander, Amelia, Martin, Mary, Edward, Anne, Catherine, and Arthur. Fortified with their bankrolls everyone begins to play Joe's system:
And voilà!
A Little Help from Joe's Friends
Note that here every single one of Joe's friends - without exception - also went broke. And there was no fiddling with the sequences here. The play of everyone in this graph is what would be seen by true random spins of the wheel. And everyone - repeat, EVERYONE - went broke playing the Martingale System.
So despite Joe's early analysis, none of the players - that's NONE! KEINS! NICHTS! - had a monotonic linear increase in winnings. Although some of the players did have an initial increase in their bankroll none so much as doubled their money. Instead eventually all the winnings showed an overall downward trend until the players lost everything.
But it's not actually the downward trend that raises eyebrows. It's the amount of the downward trend which is - as Artie Johnson might have said - verrryyyyyyy interesting.
Further elaboration will require a slight digression. Let's look what happens if Joe and his friends simply play only $1 a spin and keep track of their bankrolls. Playing at casino odds and payoff, it's clear that the casino will gradually whittle their money down.
Joe and His Friends
Playing the Minimum
Now if you do what's called a least-squares linear regression on all of the points, the line has a slope of -$0.0526 per spin. In other words, the players are losing 5.26¢ on each turn.
Joe's and His Friends
(Regression Fitting)
Students of the mathematics of gambling will immediately notice that the downward slope of 5.26¢ is the same as what gamblers call roulette's house percentage. Also called the house advantage, the rake, the juice, the cagnotte, or the vigorish ("vig" for short, a great Scrabble word), the house percentage is the average rate of loss for each bet.
The vigorish arises because in their games the casinos pay off the bets at less than correct odds. In other words, when you bet $1 on red and win, the casino pays you $1. But they should pay you $1.11.4
Footnote
The way to calculate a fair payout for a bet is from the formula:
Player's Odds
Payout($) = × Player's Bet
House Odds
... where the odds are expressed as a percentage. For a 1:1 payout bet in roulette, this ration is 10/9 or 1.11. At this payout ratio, the house advantage is not 11% as it may look but is instead the 5.26 % determined by the slope of the line.
The 5.26% house advantage applies to all the bets in roulette except what is called the 5-number combination. If you place your chip at the edge of the line separating the row with the 0 and 00 from the row with the numbers 1, 2, and 3, you are betting that the ball will land in 0, 00, 1, 2, or 3. If the ball lands in any of those slots you are paid off at 6:1 ($6 winning plus you get your $1 bet back).
The true odds, though, that any five numbers show up is 5/38. So the casino should pay off at 37 to 5 or 7.4:1. The difference between the true odds and the payoff odds gives the house its vig.
Unlike the games with complex betting like dice and blackjack, the house percentage in roulette is a fairly simple calculation. Since for the five number combination the house will lose $6 for 5/38 of the time and win $1 for 33/38 of the time, the house will come out ahead by:
| House Percentage | = | -6×5/38 + 1×33/38 | |
| = | (-30 + 33)/38 | ||
| = | 3/38 | ||
| = | 0.0789 | ||
| = | 7.89% |
So in 100 spins the house will win a bit less than 8% more often than the true odds would indicate.
Since all other bets at roulette are 5.26% against the player, betting the 5 number combination bet is often referred to as a "sucker" bet.
Now if you take the regression line and overlay it on the graph of Joe and his friends playing their system, it actually fits the data, maybe not exactly but still pretty nearly as Isaac Newton would have said. So in addition to the fact that you can't always double up, the fact that the house pays the player at less than correct odds seems to be another contributing factor in their losses.
Joe's and His Friends
System Playing
OK. The empirical evidence seems to show that Joe and his friends will eventually lose. But then how was Joe able to apparently theoretically prove to Arnold that he should win? We've always wanted to know that.
No doubt you have, as Captain Mephisto said to Sidney Brand. It's very simple really.
It's very simple.
The graph that Joe showed to Arnold was based on the idea that no how many losing spins he had in a row he had, he could always double up and so recoup his losses. But because of the betting limits, you can't always double up. So Joe's graph does not represent the real world.
And although it's not immediately obvious, Joe's theory also requires he has an unlimited bankroll. With unlimited dough, no matter how long a losing streak he has, he would always be able to bet enough to recoup his losses. But in the real world where everyone has a finite amount in the kitty - no matter how large - Joe will eventually have to bet so much that he will loose it all.
Yes, yes. We know that theoretically Joe can loose so many bets in a row that no matter how big his bankroll he'll get wiped out. But we want a realistic assessment. Not some preachy sermon on the hazards of gambling. So just how likely is it really that Joe will have those long sequence of losses in a row?
One thing about randomness is that what shouldn't happen seems to happen more often than you might think. So let's look at another betting session that is based on Joe's theory - and a session that actually does come about when performing computer - that is, the "difference engine" - simulations of playing the wheel:
| Spin | Bet ($) | Outcome | Spin Win | Bankroll ($) | Comments | 1 | 1 | WIN | 1 | 1001 | Starting Off |
| 2 | 1 | WIN | 1 | 1002 | A Win! |
| 3 | 1 | WIN | 1 | 1003 | 'Nother! |
| 4 | 1 | LOSE | -1 | 1002 | Huh! So What! |
| 5 | 2 | WIN | 2 | 1004 | Back On Track! |
| : : | : : | : : | : : | : : | Let Us Continyeh! |
| 114 | 1 | WIN | 1 | 1053 | Making the Dough! |
| 115 | 1 | LOSE | -1 | 1052 | A Loss Don't Worry! |
| 116 | 2 | LOSE | -2 | 1050 | Two Losses? Who Cares? |
| 117 | 4 | WIN | 4 | 1054 | See Won Again! |
| 118 | 1 | WIN | 1 | 1055 | And Again! |
| 119 | 1 | WIN | 1 | 1056 | And Again! |
| 120 | 1 | LOSE | -1 | 1055 | Yawn! |
| 121 | 2 | LOSE | -2 | 1053 | Nothin' to Worry About! |
| 122 | 4 | LOSE | -4 | 1049 | We'll Recoup Soon |
| 123 | 8 | LOSE | -8 | 1041 | We Think. |
| 124 | 16 | LOSE | -16 | 1025 | Hm. Gettin' Behind A Little. |
| 125 | 32 | LOSE | -32 | 993 | Still Got A Good Bankroll |
| 126 | 64 | LOSE | -64 | 929 | Gotta Win Sometime! |
| 127 | 128 | LOSE | -128 | 801 | Don't We? |
| 128 | 256 | LOSE | -256 | 545 | Something Seems Amiss! |
| 129 | 512 | LOSE | -512 | 33 | Hm. Need Another Grand. |
| 130 | 1024 | LOSE | -1024 | -991 | And Another. |
| 131 | 2048 | LOSE | -2048 | -3039 | How About Two? |
| 132 | 4096 | LOSE | -4096 | -7135 | More Than Four? |
| 133 | 8192 | LOSE | -8192 | -15327 | Sacre Bleu! |
| 134 | 16384 | LOSE | -16384 | -31711 | What The Hey? |
| 135 | 32768 | LOSE | -32768 | -64479 | This Ain't Suppose to Happen! |
| 136 | 65536 | LOSE | -65536 | -130015 | Is't Possible? (Shakespeare) |
| 137 | 131072 | LOSE | -131072 | -261087 | Deep Pockets Needed! |
| 138 | 262144 | LOSE | -262144 | -523231 | Augh! Not Again? |
| 139 | 524288 | WIN | 524288 | 1057 | No Sweat Back in Action! |
| : : | : : | : : | : : | : : | : : |
| 1998 | 1 | WIN | 1 | 1999 | Whew! |
| 1999 | 1 | LOSE | -1 | 1998 | Well, One More Win And It's A Day |
| 2000 | 2 | WIN | 2 | 2000 | Doubled The Bankroll! |
But as a picture is worth a thousand words, look what we see if we summarize this session in a nice neat graph.
Unlimited Bankroll
So yes, in our example Joe could recoup his losses. But he would have had to go to Arnold and borrow over half a million smackers to do so. Needless to say Joe didn't show this graph to Arnold.
Again the curmudgeons object. It's all well saying you see a long string of losses more than you expect. But c'mon! In this - quote - "example" - unquote - Joe loses 19 times in a row, for crying out loud! How likely is that?
Now odds makers tells us this will happen only about once in every two hundred thousand spins of the wheel, for Pete's sake! What we need to know, though, is just how many successive losses can Joe expect in a typical session at the wheel and how much can he expect to lose?
Well, to answer this question we need to first calculate the expectation value - that is, the average number - of consecutive losses. That is, we ask what will be the average number of sequential black and green numbers (0 and 00) with no red numbers?
First of all, the probability that any spin will be a losing spin of length 1 is simply 20/38. That's just the probability that you will lose a single bet. This probability is simply the sum of the 18 black numbers and the two green numbers divided by the total numbers appearing the wheel.
So the odds of Spin #1 being a "run" of losses of length 1 is:
P1 = (18 + 2)/38
= 20/38
= 0.526
... or 52.6%.
OK. What about getting two losses in a row? Well, that's the probability of getting one loss in a row followed by another loss. So you multiply the probability of one loss by 20/38.
P2 = (20/38)(20/38)
= 400/1444
= 100/361
And for other successive losses we keep up the process:
| Probabilities of Loss (Black or Green) | ||
| Consecutive Losses | Probability | |
| 1 | 20/38 | 0.444 |
| 2 | (20/38)2 | 0.296 |
| 3 | (20/38)3 | 0.146 |
| : | : | : |
| n | (20/38)n | |
So in general the probability that you'll have a run of losses of length i is:
P(i) = (20/38)i
Now we need to cite another and well known rule of mathematics. And that's for any statistical property (or random variable), X, the expectation value of the property, X, is the sum of the product of all possible values of the property at value i, Xi, times the probability of that Xi will occur, PXi.
All Possible
Values
X = Σ XiPXi
i=1
Now note that in our case, there is no limit to the number of spins, (i). That is, all possible values is infinity (∞). So we multiply the length of the run with the probability of that length and sum them up over infinity and we get the expectation value of the length of the losing runs - which we call L - is:
∞
L = Σ i(20/38)i
i=1
... which might be a little more comprehensible if the summation is expanded as:
L = (1)(20/38)1 + 2(20/38)2
+ 3(20/38)3 + ...
Now at this point we can pause and look at some specific examples of the sums.
| L (Expected Length of Losses) | ||||
| n |
|
|||
| 1 | 0.526 | |||
| 2 | 1.080 | |||
| : | : | |||
| 5 | 1.518 | |||
| 6 | 2.154 | |||
| : | : | |||
| 10 | 2.324 | |||
| : | : | |||
| 20 | 2.346 | |||
| : | : | |||
| 50 | 2.346 | |||
| : | : | |||
| 100 | 2.346 | |||
| : | : | |||
| 200 | 2.346 | |||
Hm. Most interesting. It seems that as you spin the wheel more and more, the expectation values of sequential losses, L, hits a constant number. That is, the summation converges. So the average number losses before you get a win is a rather small value.
OK. But these are merely specific examples of average sequential losses for a finite number of spins. Is it possible that if we continue with more spins eventually the numbers do get larger and larger? Is it possible that the average number of consecutive losses is eventually infinite?
So we really DO need to calculate the sum over all possible values.
True it's rather tedious - and actually impossible - to individually sum up an infinite number of terms. Instead we introduce the notion of the partial sum of a series.
A partial sum is a formula that gives us the total sum of a given number of terms in a series in one nice neat formula. For instance, suppose you have the summation:
n
Sn = Σ i
i=1
... which is:
Sn = 1 + 2 + 3 + 4 + ... + n
Clearly summing up a lot of numbers, say the first 65,537 positive integers, would be a rather tedious task. But the formula for the partial sum of this series is simply:
n(n + 1)
Sn =
2
So the sum of the number from 1 to 65,537 is:
n(n + 1)
Sn =
2
65,537(65,537 + 1)
=
2
4,295,163,906
=
2
= 2,147,581,953
Now if we have the summation:
n
Sn = Σ iai
i=1
... which can be expanded as:
Sn = a + 2a2 + 3a3 + 4a4... + iai
This, in turn, is similar to a famous series:
Sn = 1 + x1 + 2x2 + 3x3... + nxn
... which with a little middle school math can be shown to have the partial sum:
nx(n + 1) - (n + 1)xn + 1
Sn = x + 1
(x - 1)2
Now notice that if we let 20/39 = a = x then our original series is:
Sn = x + 2x2 + 3x3 + 4x4... + ixi
... which is 1 less than the series where we have the partial sum. Conveniently the partial sum we just calculated has the number 1 at the end. So we just lop that off and the partial sum of our series is:
nx(n + 1) - (n + 1)xn + 1
Sn = x
(x - 1)2
Remember that we want to calculate the sum of all possible terms, and in our case that's an infinite number of terms. And to calculate the sum of the infinite number of terms we must take the limit of the partial sum as n goes to infinity.
Now delving into a textbook of calculus we learn that:
lim (n + 1)an = 0 if 0 < a < 1
n→∞
And that:
lim nan = 0 if 0 < a < 1
n→∞
... which fits our series where x = a =20/38
So with minimalistic algebra we have:
x
Sn=∞ =
(x - 1)2
Putting the numbers in the formula for the partial sum, we have:
20/38
Sn=∞ =
(20/38 - 1)2
... and ...
20/38
Sn=∞ =
(-18/38)2
... which is:
Sn=∞ = 190/81
... and hey, presto!, we know that Sn=∞ is the same as our expected lenght of losses in a row, , L, and by calculating the value of 190/81 we have:
L = 2.345679
... which fits the numbers in the previous table.
So the average number of times you'll lose in a row is between 2 and 3 spins of the wheel. It's left as an exercise for the reader to show that the average number of wins in a row is 341/200 = 1.71.
Well, say the optimists, with only 2 to 3 losses expected in a row, does that mean we can expect to lose less than $10 before we win? So our bankroll of $1000 oughta be plenty to tide us over.
Sounds good.
Except that Joe and his friends all lost their shirts.
"I understand"
- Manhunt of Mystery Island, Republic, 1945
OK. We've seen the average number of losses in a row is between 2 and 3 spins. That's the expectation value of the number of sequential losses.
But what is the actual expectation value of the amount of money lost due to the expected value of the sequential losses? Wasn't the guess that this shouldn't be much more than $10?
Well, that's the guess. But that doesn't mean it's correct.
So once more we'll use the partial sums of losses. Then we'll look at the average amount of money lost before winning the next dollar.
OK. Remember the probability of a given number of losses in a row i is:
Pi = (20/38)i
Also remember that you double the amount you last bet when you lose. So the doubled bet times the probability of the number of losses:
Pi = 2i(20/38)i
A little more algebra gives us:
Pi = (2 × 20/38)i
So the summation of the first n spins is:
n
Loss = Σ (2×20/38)i
i=1
... and this is:
n
Loss = Σ (40/38)i
i=1
n
= Σ (20/19)i
i=1
Hm. This looks familiar. It is in fact our old friend.:
n
Si = Σ xi
i=1
... where x = 20/19.
So doesn't this summation also converge nice and neatly to a small number?
Well, we must be honest and say:
 NO!
NO!
Eins mehr let's make a table of the data. Remember we don't use the formula for the infinite summation but we have to use the formula for the finite partial sums which is:
nx(n + 1) - (n + 1)xn + 1
Sn = x
(x - 1)2
... where x = 20/19.
So putting the right numbers in the right place we end up with:
| Average (Expected Loss) | ||
| n | Expected Run Length | Expected Loss Before Win ($) |
| 1 | 0.526 | 0.526 |
| 2 | 1.08 | 1.63 |
| : | : | : |
| 5 | 2.03 | 9.07 |
| : | : | : |
| 10 | 2.32 | 39.68 |
| : | : | : |
| 50 | 2.35 | 4218.85 |
| : | : | : |
| 100 | 2.35 | 137002.09 |
| : | : | : |
| 200 | 2.35 | 51636775.56 |
| : | : | : |
Sacrebleu! (to quote Zola). How can such a formula which earlier produced a nice small and convergent number keep going up? Est-ce possible?
Well, it is pose-ee-blay. Remember that our partial sum converged only if the limits of (n + 1)an and nan both converge to 0. But that happens only if a < 1.
But when calculating the money lost, a = 20/19. This means a = 1.053 - which is bigger than 1. So as i gets bigger (which sounds like horrible grammar) this new summation grows without limit. That is the summation "blows up" as the mathematicians say.
Remember one guess was that since the since the average number of losses between wins is only around 2.34, we wouldn't even lose as much as $10 before we recoup our losses. But according to the actual numbers we're going to lose a lot more than that.
How much? Well, take the limit of the formula for partial sums where n goes to infinity.
n(20/38)(n + 1) - (n + 1)(20/38)n + 1
Sn=∞ = lim (20/38)
n→∞ ([20/38] - 1)2
And without going into tedious details we can prove:
n(20/38)(n + 1) - (n + 1)(20/38)n + 1
Sn=∞ = lim (20/38)
n→∞ ([20/38] - 1)2
= ∞
... which means that for a sequence of losses - and pardon us if we shout -
 THE
THE
AVERAGE EXPECTED
LOSS FOR THE
MARTINGALE SYSTEM
IS
INFINITE!
Which means you can expect to loose everything.
"If you shall fright me into labor and concentration,
I shall win my game."
- Ralph Waldo Emerson, The Correspondence of Thomas Carlyle and Ralph Waldo Emerson.
OK. We can loose it all. But how much can you win?
Well, that's easy. If you played the Martingale System and it was possible to always double your bet, once you do win you'll recoup your losses and come out $1 ahead. So the expectation value of winning following a sequence of losses is always $1.5
Footnote
The $1 expectation value can be proven using the summation of the probabilities as before. The probability of having a win regardless of the number of previous losses is simply(18/38)(20/38)(i - 1) where i is the number of spins. Since the sequences is always followed by a win of $1, the expected winning is
| n |
| Σi(18/38)(20/38)(i - 1) |
| i=1 |
This can be proven using one of the partial sum formulas given above plus knowing that if you have a summation where each term has a constant you can move the constant outside of the summation sign.
So as Flakey Foont asked Mr. Natural, what does it all mean? Well, it's not Mr. Natural's famous reply. It does mean that with using our betting strategy, then
 THE
THE
EXPECTED
MONETARY
RISK
FOR THE
MARTINGALE SYSTEM
TO WIN
$1
IS
INFINITE.
These are not good odds.
And In Conclusion
- William Shakespeare
Now let's say it out loud and all together:
 A
A
NECESSARY
CONDITION
FOR THE
MARTINGALE SYSTEM
TO WORK
IS AN
UNLIMITED
BANK ACCOUNT.
... and ...
 A
A
NECESSARY
CONDITION
FOR THE
MARTINGALE SYSTEM
TO WORK
IS
NO BETTING LIMITS.
This does not happen if we're in the real world.
But you say even if - as the Spartans told Phillip - Joe can't sit at the table with an infinite amount of money to cover the losses he can have someone slip him an extra grand if his initial bankroll goes bust. So is it possible that least in principle he can keep on playing?6
Footnote
The possibility of having unlimited borrowing power illustrates one the long-standing questions in the philosophy of mathematics. This is the difference between a set with an infinite number of elements or a set with a potentially infinite set of numbers.
A set with an actual infinity of elements is a set like the number of points between 0 and 1. Surely there is no limit to the points but all the points are there on the line.
But for something like a bankroll it cannot be an actual infinity because there is never an infinite amount of money anywhere. Not in a bank, not in a country, and not in the universe.
However it is possible to always make more currency. So even if you borrow all the money that's currently in the universe, you could still print up more cash. The cash availble is an example of a potentially infinite set. However, in practice there are actually limits to that as well.
Well, suppose we have to live with the betting limit but even if we don't exactly have an unlimited or infinite bankroll, we have a friend who will alway lend us another grand if we go bust.
We then we start to bet. We also keep tabs, not just of our bankroll which is being replenished if necessary, but our total winnings. That is, how much dough we have taken from the casino.
And what we find is:
Joe's Replenished Bankroll
Betting Limits: $1 to $100
Hm. The amount we "win" is a negative number - which mean we're losing - and our "winnings" keep going down - which means our losses get bigger! So even if Joe has the potentially unlimited bankroll where he can go to replenish his losses, he doesn't really win. As he keeps playing, his losses - and his debt to his benefactor - get bigger and bigger.
A Most Amusing Paradox
- Gilbert and Sullivan (The Pirates of Penzance)
In the end the Martingale system comes off as something of a paradox. In an imaginary world with no betting limits and an infinite bankroll - you can hypothetically turn a profit even if the payout is at casino odds. But in the real world, the combination of having a finite amount of money and betting limits - not to mention the House's vigorish - means you'll eventually go broke.
But it's never good to leave on a negative note. We can always find a few bon mots suitable for the topic.
| Why do zoo animals never gamble? | Because there's always some cheetahs. |
| Why are dietitians never allowed at blackjack tables? | They're carb counters. |
| What book did John Milton write about gambling? | Pair-a-Dice Lost. |
| Why do people never go to southern France to gamble. | No one like Toulouse. |
... and finally there's:
| How can you leave Las Vegas with a hundred grand? | Go there with half a million. |
References and Further Reading
The Mathematics of Games and Gambling, Edward Packel, The Mathematics Association of America, 1981.
Understanding Probability: Chance Rules in Everyday Life, Henk Tijms, Cambridge University Press, 2004, (Third Edition, 2012).
Scarne's New Complete Guide to Gambling, John Scarne, Simon and Schuster, First Edition (1961), Second Edition (1974).
The Big Bankroll, Leo Katcher, Harper Brothers, 1958.
The Unsinkable Titanic Thompson, Carlton Stowers, Eakin Press, 1982.
The Odds Against Me, John Scarne, Doubleday, 1966.
The Weekend Gambler's Handbook,, Major Riddle, Random House, New York, 1963.
An Introduction to the Bootstrap, Bradley Efron and R.J. Tibshirani, Chapman and Hall/CRC Monographs on Statistics and Applied Probability, 1993.
Emile or On Education, Jean-Jacques Rousseau, Amsterdam Néaulme, 1762.
Maxims for Revolutionists, George Bernard Shaw, Archibald Constable and Company, Ltd., 1903.
The Correspondence of Thomas Carlyle and Ralph Waldo Emerson, 1834-1872, Thomas Carlyle and Ralph Waldo Emerson, Houghton-Mifflin, 1883 - 1884.
"When the U.S. Air Force Discovered the Flaw of Averages", Todd Rose, Toronto Star, January 16, 2016.
De Garrulitate, Plutarch, Perseus, Tufts University.
Diamonds Are Forever, Ian Fleming, Jonathan Cape, 1959.